Tuesday, June 7, 2011
Monday, May 23, 2011
Unit 5- Scientific Notation
SUMMARY OF KNOWLEDGE
The purpose of scientific natation is make numbers easier to work with.
EX. 12,000,000,000=1.2*10^9
with scientific notation we can represent and display really large and small numbers easier
KEY VOCABULARY
Scientific Notation= a short way to write very large or very small numbers.
Standard Notation= the most common way of writing numbers.
EX. 254= 2 hundreds 5 tens 4 ones
TASK ANALYSIS
Standard-Scientific
make the number smaller than 10 but bigger than 1 and put the number of zeros on the exponent and *10 before the exponent
EXAMPLES
1. Standard-Scientific
with scientific notation we can represent and display really large and small numbers easier
KEY VOCABULARY
Scientific Notation= a short way to write very large or very small numbers.
Standard Notation= the most common way of writing numbers.
EX. 254= 2 hundreds 5 tens 4 ones
TASK ANALYSIS
Standard-Scientific
make the number smaller than 10 but bigger than 1 and put the number of zeros on the exponent and *10 before the exponent
EXAMPLES
1. Standard-Scientific
2,500,000=2.5*10^5
2. Scientific-Standard
2.5*10^5= 2,5+0+0+0+0+0= 2,500,000
3. Negative Scientific to Standard
2.5*10^-5= 0+0+0+0+2.5= .000025
2.5*10^-5= 0+0+0+0+2.5= .000025
Useful Links
http://www.math.toronto.edu/mathnet/plain/questionCorner/scinot.html
http://www.mathace.net/misc/scientific-notation
http://science.widener.edu/svb/tutorial/scinot.html
Unit 6 - Making Nets
Summary of knowledge:
The goal for this project is to make a blog explaining people how to de nets wich is our theme, students should understand the concept of a net and how to do it for rectangular prisms.
The goal for this project is to make a blog explaining people how to de nets wich is our theme, students should understand the concept of a net and how to do it for rectangular prisms.
Unit 4 - Extending the Number Line
Summary of Knowledge:
Students should identify negative and positive numbers.
Students should identify the value of positive, negative, and rational numbers in order to place them on a number line.
Students should be able to know the relationship between positive or negative numbers and their opposites.
Key Vocabulary:
Negative Numbers: A number less than 0. On a number line, negative numbers are located to the left of 0. On a vertical number line, negative numbers are located below 0.
Positive Numbers: A number greater than 0. 0 is neither positive nor negative. On a number line, positive numbers are located to the right of 0. On a vertical number line, positive numbers are located above 0.
Opposites: Two numbers whose sum is 0. For example: -3 and 3 are opposites. On a number line, opposites are the same distance from 0, but in different directions from 0. The number 0 is its own opposite.
Integers: The whole numbers and their opposites. 0 is an integer, but is neither positive nor negative.
Rational Numbers: numbers that can be expressed as a quotient of 2 integers where a divisor is not 0.
Task Analysis of how to Place Numbers on the Number Line:
Examples :
Kate and brad have a project of measuring the temperature in the Sahara desert on their new film. They recorded these temperatures : 40 , 20 , 0 , -20 , -40. Do a number line and plot the temperatures in it.
Juanes is doing a number line and which he has to record the numbers : -2,0,2,4,-4. Do a number line , and plot the numbers.
Web References :
Web References :
http://www.helpingwithmath.com/resources/oth_number_lines.htm
www.funbrain.com/linejump/index.html
www.mathsisfun.com/number-line.html
By Juan Andres Zepeda and Nicolas Chavez
Unit 5 - Exponent Rules
Summary of knowledge:
The goal for this investigation was to understand exponents and learn how to use them. By completing this unit students shoul understand why and how the exponents work and the task analysis or algorithms to solve a problem.
Key Vocabulary:
Base - The face of a 3-Dimensional shape, chosen to be the bottom base.
Exponent - A symbol or number placed above and after another symbol or number to denote the power in which later is to be raised.
Task Analysis:
Multiplication Properties of Exponents:
1) Product of Powers property
To multiply powers having the same base, add the exponent.
In General: a^m*a^n=a^m+^n
1) Quotient of Powers Property
To divide powers having the same base, subtract exponents.
In General: a^m/a^n=a^m-n
Ex:
1. 6^5/6^4=6^1=6
2. 9^4*9^2/9^7=9^6/9^7=9^-1
3. (-5^2)/(-5^2)=(-5^0)=1
4. y^3/y^5=y^-2
2) Power of a Product or Quotient Property:
To find a power of a product or quotient, distribute the exponent over the factors.
In General: (a*b)^m=a^m*b^m
(a/b)^m=a^m/b^m
Ex:
1. (6*5)^2=6^2*5^2
2. (4yz)^3=4^3*y^3*z^3
3. (2/3)^2=2^2/3^2
4. (7/4)^-3=7^-3/4^-3=4^3/7^3
http://www.algebralab.org/lessons/lesson.aspx?file=Algebra_ExponentsRules.xml
The goal for this investigation was to understand exponents and learn how to use them. By completing this unit students shoul understand why and how the exponents work and the task analysis or algorithms to solve a problem.
Key Vocabulary:
Base - The face of a 3-Dimensional shape, chosen to be the bottom base.
Exponent - A symbol or number placed above and after another symbol or number to denote the power in which later is to be raised.
Task Analysis:
Multiplication Properties of Exponents:
1) Product of Powers property
To multiply powers having the same base, add the exponent.
In General: a^m*a^n=a^m+^n
Examples:
1. 3^2*3^7= 3^2+^7=3^9
2. 3*3^5=3^1+^5=3^6
3. (-2)^2+(-2)^4=(-2)^6
4. x^2*x^3*x^4=x^9
2) Power of a Power Property
To find a power of a power,multiply the exponent.
In General: (a^m)^n=a^m*^n
Examples:
1. (5^2)^4=5^2*4=3^8
2. (y^3)^4=y^12
3. [(y^3)^3]^2=-3^6
2. 3*3^5=3^1+^5=3^6
3. (-2)^2+(-2)^4=(-2)^6
4. x^2*x^3*x^4=x^9
2) Power of a Power Property
To find a power of a power,multiply the exponent.
In General: (a^m)^n=a^m*^n
Examples:
1. (5^2)^4=5^2*4=3^8
2. (y^3)^4=y^12
3. [(y^3)^3]^2=-3^6
Division Properties of Exponents
1) Quotient of Powers Property
To divide powers having the same base, subtract exponents.
In General: a^m/a^n=a^m-n
Ex:
1. 6^5/6^4=6^1=6
2. 9^4*9^2/9^7=9^6/9^7=9^-1
3. (-5^2)/(-5^2)=(-5^0)=1
4. y^3/y^5=y^-2
2) Power of a Product or Quotient Property:
To find a power of a product or quotient, distribute the exponent over the factors.
In General: (a*b)^m=a^m*b^m
(a/b)^m=a^m/b^m
Ex:
1. (6*5)^2=6^2*5^2
2. (4yz)^3=4^3*y^3*z^3
3. (2/3)^2=2^2/3^2
4. (7/4)^-3=7^-3/4^-3=4^3/7^3
For more help visit:
http://www.algebralab.org/lessons/lesson.aspx?file=Algebra_ExponentsRules.xml
Unit 6 - Surface Area and Volume for Rectangular Prisms
Summary of Knowledge:
The goal of this investigation is to be able to create a net, be able to create a box, and create net with given bow or create a box with a given net.This is very important because it may help us in the future specially if we decide to become architects. The main key terms that appear in this section are Surface Area and The Volume of a rectangular prism.
Key V0cabulary:
cube: A three-dimensional shape with six identical square faces
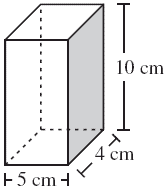
The goal of this investigation is to be able to create a net, be able to create a box, and create net with given bow or create a box with a given net.This is very important because it may help us in the future specially if we decide to become architects. The main key terms that appear in this section are Surface Area and The Volume of a rectangular prism.
Key V0cabulary:
cube: A three-dimensional shape with six identical square faces
8 vertice
- 12 edges
net: A net is a two-dimensional shape pattern that can be folded to form a three-dimensional shape.
rectangular prism: A prism with a top and a bottom that are congruent rectangles.
base: The face of the three-dimensional shape chosen to be the bottom face
Task Analysis:
Suface Area: first you must find the are of each face of the rectangular prism. Then you add them up and you have the surface area. You could also find the surface area by getting the area of the three distinct faces and then multiply them by two.
Volume:to find the volume of a rectangular prism yopu must multiply the dimensions.
The equation for finding the volume of a rectangular prism is (lxwxh)
Examples:
The volume of this box is 8 sinse the dimensions are 2x2x2 (lxwxh)
The surface are of this cube 12 since the dimension of an face is 2x2=4 sinse its a cube you multiply times 6 that equals 12.
Unit 5 - Square and Cube Roots
Summary of Knowledge:
The goal for this investigation is to use square roots to find the side lengths of a square using the given area. You know that a square has two same sides and multiply both to give you thre area (x^2 ) (x*x)
Cube roots are important to find the side lengths of a cube by a given area.
Ex: volume : 27
side length: 3
( 3*3*3 = 27)
Key Vocabulary:
Irrational Number: A number in the decimal portion that never ends and does not repeat.
Cube roots: A number that must be multiplied by itself three times to equal a given number.
Ex: 27 the cube roots of 27 is 3 (3*3*3)
Square Roots: A nonnegative numner that must be multiplied by itself to equal a given number.
Ex: 4 the square root of 4 is 2 (2*2)
Task Analysis Square Roots:
1 Numbers should be perfect squares. This means that the product of a number multiplied by itself ( use nultiplication tables to help you know which numbers are square roots.)
2 If a number is not a square number write the two square roots in between.
Ex:
√20 Irrational in between 4 and 5
4*4 =16 5*5=25
20 is in between 16 and 25
Task Analysis of Cube Roots
1: Think of 2 factors that make the number that you are finding the cube roots.
Ex:
∛8
Factors: 2 AND 4
2: Then see if the small number can be used to divide the big number( the answer has to be the same as the small number). Also you can think if the big number is a square number. And of the square root is the small number.
Ex:
∛8 Factors: 2 AND 4
Factors of 4 : 2, 2 (2x2= 4)
3:Then multiply the 3 small numbers and the number should be the cubed number in the beggining.
Ex: 2x2x2 = 8 ( the cubed root of 8= 2)
4:If the number its irrational think of two numbers that are in between the cubed number.
Ex:
∛16
The 2 cube numbers are: 8 and 27
The cube root f 8: 2 (2x2x2) The cube roots of 27 : 3 (3x3x3)
The answer is : irrational in between 2 and 3
Examples of Square and Cube roots
∛27:= 3 this means that 3x3x3=27
√30 = irrational in between 5 and 6
Take this quiz to test your knowledge : if you need more help go to this useful links below.
style="FONT-SIZE: 10px; COLOR: #000; FONT-FAMILY: Arial, Helvetica, sans-serif">Square and Cube Roots » Make A Quiz
Very Useful Links!!!!
Square roots: http://www.ixl.com/1f?gclid=CK7-xbXcsakCFced7QodjWUoKQ
Cube Roots: http://www.onlinemathlearning.com/cube-roots.html
Unit 4 - Adding and Subtracting
Summary of Knwledge:
Our goal is that students will learn how to add and subtract positive and negative numbers. Learn how to do algorithms for calcualete the sum and differences, and how to use a number line to help the adding and subtracting.
Key Vocabulary:
Commutative Property: The order of the addition or multiplication of two numbers does not change the result.
Absolute Vlue: the absolute value of a numbers is the distance from zero on a number line. Simbolysed by (n).
Task Analysis:
1. See if the numbers are negative or positive.
2. See were are the numbers are located in number line.
3. convetr 2 doble sign numbers to just one sign.
+ + = +
+ - = -
- + =-
- - = +
4. Count the numbers of spaces if is adding to the right and to left is subtracting.
Examples:
Quiz:
Ading and Subtracting Quiz » quiz
Video Exapmles:
Images that will help you!!!
How to change double sign to a
simple sign.
Helpful Web Pages:
http://www.shodor.org/interactivate/activities/ArithmeticFour/
Our goal is that students will learn how to add and subtract positive and negative numbers. Learn how to do algorithms for calcualete the sum and differences, and how to use a number line to help the adding and subtracting.
Key Vocabulary:
Commutative Property: The order of the addition or multiplication of two numbers does not change the result.
Absolute Vlue: the absolute value of a numbers is the distance from zero on a number line. Simbolysed by (n).
Task Analysis:
1. See if the numbers are negative or positive.
2. See were are the numbers are located in number line.
3. convetr 2 doble sign numbers to just one sign.
+ + = +
+ - = -
- + =-
- - = +
4. Count the numbers of spaces if is adding to the right and to left is subtracting.
Examples:
Quiz:
Ading and Subtracting Quiz » quiz
Video Exapmles:
Images that will help you!!!
How to change double sign to a
simple sign.
Helpful Web Pages:
http://www.shodor.org/interactivate/activities/ArithmeticFour/
Unit 4 - Multiplying and Dividing
Summary of Knowledge:
The goals of this investigation is to develop algorithms for multiplying and dividing. You will also notice number patterns. You will learn multiplication and division of integrers, to answer questions about speed, time, distance and direction of motion. Upon the completition of this investigation you will be able to divide and multiply negative numbers.
Key Vocabulary:
1. Commutative Property: the order of the addition or multiplication of
2 numbers does not change the result.2. Absolute value: the absolte value of a number is its distance from zero in a number line it is written (-4)
Task Analysis:
Multiplication and Division:
1. When you multiply/divide a negative number with another negative number your answer will be a positive.
2. When you multiply/divide an odd number of negatives, your answer be a negative number.
3. When you multiply/divide an even number of negatives, your answer will be a positive number.
Absolute Value:
1. If the number is a positive, the absolute value will be the same number because it is the distance from 0.
2. If the number is a negative, the absolute value will be the same number but positive.
Examples:
Multiplication : a. 15 x -5 = -75 c.1/8 x -3/4 =-3/32
b. -10x -7= 70 d. -5/10 x -8/10= 40/100
Division: a. -24 ÷ 6= -4 b. -35÷-5=7
c. -36/40 ÷ -2/4 =
-36/40x -4/2= 144/80 d. 4/5 ÷ -10/15=
4/5 x -15/10= -60/50
Absolute Value:
–3 = 3
Multiplying and Dividing Negatives Quiz! » Quiz maker software
Unit 6 - Surface Area and Volume for Cylinders
Summary of Knowledge:
The goals of this investigation are to learn how to find the surface area and volume of a cylinder, and we should know the formulas to find the surface area and volume of a cylinder.
Key Vocabulary:
Cylinder: A three-dimensional shape with a top and a base that are congruent circles.
Task Analysis:
The goals of this investigation are to learn how to find the surface area and volume of a cylinder, and we should know the formulas to find the surface area and volume of a cylinder.
Key Vocabulary:
Cylinder: A three-dimensional shape with a top and a base that are congruent circles.
Task Analysis:
- To find the surface area of a cylinder you should first find the radius, then multiply it by itself .(r*r), times Pi (3.14) times two: 2*(r*r*3.14) You have to multiply it by two because they are congruent circles.
- And then add the height times two times Pi (3.14) times radius (this is the area of the lateral rectangle)
- So it will be: 2* (r*r*3.14) + height *2 *3.14 *radius. That will be the surface area.
- To find the volume of a cylinder you should multiply radius times radius times 3.14 times the height
- We have to find the area of the base and then multiply it by the height.
Examples:
The diameter of a cylinder is 10 cm, and the height is 8 cm. What is the surface area and the volume?
volume:5*5*3.14*h=628.31 cm3
surface area:5=radius(1/2 of diameter) 5*5*3.14*2=157.07+8*10*3.14=408.40 cm2
helpful webs
Subscribe to:
Posts (Atom)